Mathematics – Page 6 – E. Kowalski's blog

AMS Open Math Notes

When I was attending the conference in honor of Alex Lubotzky’s 60th birthday, Karen Vogtmann, who was also there, told me of the Open Math Notes repository, a new project of the AMS that she was involved with. This is meant to be a collection of (mostly) lecture notes, such as many mathematicians write for a course, but which are not published (nor necessarily meant to be published). So they can be incomplete, they might contain mistakes, and may more generally be subject to all the slings and arrows that mathematical writing is heir to. (See the web site for more information, submission guidelines, etc…)

I think that this is a great idea, and am very happy that, as the web site is now public, two of my own lecture notes can be found among the inaugural set! The highlight of the current selection is however undoubtedly “A singular mathematical promenade”, by Étienne Ghys, his beautiful book on graphs of polynomials, Newton’s method, Puiseux expansions, divergent series, and much much else that I have yet to see (I’m only one-third through looking at it…)

Hopefully, the Open Math Notes collection will grow to contain many further texts. The example of the book of Ghys is already an illustration of how useful this may be — although it is also available on his home page, one doesn’t necessarily visit it frequently enough to notice it…

Two final whimsical remarks to conclude: (1) among the six authors currently represented [Update (four hours later): this has already changed!], three [Update: four] (at least) are French; (2) one of my set of notes promises a randonnée, and Ghys’s book is a promenade — clearly, one can think of mathematics as a journey…

Unicyclic groups

When working on large finite field versions of conjectures like Bunyakowski’s or Schinzel’s or Bateman-Horn’s, one small point that always somewhat tickled me was that the answer, which follows from the computation of some Galois group, seems to be weaker than this actual computation. For instance, to get the “right” answer in Bunyakowski’s Conjecture, one computes a Galois group $G$ that is in a natural way a subgroup of the symmetric group on $n$ letters, and it is enough to show that the proportion of $n$ -cycles in $G$ is exactly $1/n$ . It is of course sufficient to show that $G$ is the full symmetric group (which contains the right number of $n$ -cycles), but a priori, this is a weaker condition.

Although I mentioned this briefly in my talk in the recent conference in honor of A. Lubotzky’s 60th birthday (where I was greatly honored to be invited!), it’s only last week or so that I somehow finally did the obvious thing, namely some experiments with Magma to see if the property of having proportion $1/n$ of $n$ -cycles is widespread.

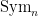When doing this, the first thing to realize (which I only did when P.P. Palfy pointed it out during that conference…) is that this condition, for a subgroup $G$ of $\mathrm{Sym}_n$ , is equivalent with $G$ containing a unique conjugacy class of $n$ -cycles (simply because the centralizer of an $n$ -cycle, either in $G$ or in the bigger symmetric group, is the cyclic subgroup of order $n$ that it generates). So we can coin a solid decent name for these groups: we call them the unicyclic permutation groups.

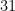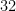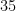Magma has a database of all transitive permutation groups of degree up to $31$ (even $32$ if one installs an extra specific database). Experimentation shows that there often exist unicyclic groups that are not the symmetric group. For instance, for degree $20$ , there exist (up to isomorphism) $1117$ transitive permutation groups, and $332$ of them contain at least one $20$ -cycle, and $35$ of them are unicyclic.

However, the same experiments show that if we restrict our attention to primitive subgroups, then the situation is very different: either there is only the symmetric group 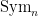$\mathrm{Sym}_n$ , or there are two unicyclic groups. Amusingly the second case occurs if and only if $n$ is prime (an amusing primality test…)

One can indeed prove that these facts (which I did first experimentally notice) are correct, but it is not cheap. More precisely, from the Classification of Finite Simple Groups, Feit and later Jones (see for instance this paper which has a slightly more general result) deduced a full classification of primitive permutation groups that contain an $n$ -cycle. 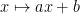It is restrictive enough that one can then relatively easily exclude all groups except the symmetric groups and, when $n$ is prime, the group of transformations $x\mapsto ax+b$ of the finite field $\mathbf{F}_n$ .

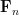That’s a nice result, but is it relevant for our original motivation? I think so, because (as Will Sawin pointed out), one can prove in considerable generality that the Galois group in (say) the Bunyakowski problem is primitive, before or without computing it exactly, because it suffices to prove that it is 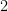$2$ -transitive, and this is accessible to the diophantine interpretation using Chebotarev’s Density Theorem, as in Entin’s approach. (Indeed, Entin goes on to check that the Galois group is often $7$ -transitive or so, and from the Classification of Finite Simple Groups, deduces that the group contains the alternating group). 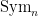Hence, if the degree $n$ is not prime, granted that one first applies Entin’s method to check primitivity, having Galois group $\mathrm{Sym}_n$ is equivalent to the natural version of Bunyakowski’s conjecture in the large finite field limit…
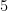If $n$ is prime, well one can say that if $G$ is not solvable, then it must be the symmetric group (assuming $n$ is at least $5$ …), although that is not as good — is there a better way to do it?

Symmetric powers and the Chinese restaurant process

Here is a simple but cute computation that makes a good exercise in elementary representation theory with a probabilistic flavor — as usual, it’s probably well-known, but I wasn’t aware of it until doing the exercise myself.

We consider random permutations in the symmetric group $S_n$ , and write $\omega_n(\sigma)$ for the number of cycles in the disjoint cycle representation of $\sigma\in S_n$ . It is a well-known fact (which may be due to Feller) that, viewed as a random variable on $S_n$ (with uniform probability measure), there is a decomposition in law
$\omega_n=B_1+\cdots+B_n,$
where $B_i$ is a Bernoulli random variable taking value $0$ with probability $1-1/i$ and $1$ with probability $1/i$ , and moreover the $(B_i)$ ‘s are independent.

This decomposition is (in the sources I know) usually proved using the “Chinese Restaurant Process” to describe random permutations ( $n$ guests numbered from $1$ to $n$ enter a restaurant with circular tables; they successively sit either at the next available space of one of the tables already occupied, or pick a new one, with the same probability; after all $n$ guests are seated, reading the cycles on the occupied tables gives a uniformly distributed element of $S_n$ .) If one is only interested in $\sigma_n$ , then the decomposition above is equivalent to the formula
$\mathbf{E}(z^{\omega_n})=\prod_{j=1}^n\Bigl(1-\frac{1}{j}+\frac{z}{j}\Bigr)$
for the probability generating function of $\omega_n$ . (This formula comes up in mod-poisson convergence of $\sigma_n$ and in analogies with the Erdös-Kac Theorem, see this blog post).

Here is an algebraic proof of this generating function identity. First, $z\mapsto \mathbf{E}(z^{\omega_n})$ is a polynomial, so it is enough to prove the formula when $z=m$ is a non-negative integer. We can do this as follows: let $V$ be a vector space of dimension $m$ over $\mathbf{C}$ . Then define $W=V^{\otimes n}$ and view it as a representation space of $S_n$ where the symmetric group permutes the factors in the tensor product. Using the “obvious” basis of $W$ , it is elementary that the character of this representation is the function $g\mapsto m^{\sigma_n(g)}$ on $S_n$ (the matrix representing the action of $g$ is a permutation matrix). Hence, by character theory, $\mathbf{E}(m^{\sigma_n})$ is the dimension of the $S_n$ -invariant subspace of $W$ . Since the representation is semisimple, this is the dimension of the coinvariant space, which is the $n$ -th symmetric power of $V.$ This in turn is well-known (number of monomials of degree $n$ in $m$ variables), and we get
$\mathbf{E}(m^{\omega_n})=\binom{m+n-1}{n}=\prod_{j=1}^n\Bigl(1-\frac{1}{j}+\frac{m}{j}\Bigr),$
as claimed!

Update on a bijective challenge

A long time ago, I presented in a post a fun property of the family of curves given by the equations
$x+1/x+y+1/y=t,$
where $t$ is the parameter: if we consider the number (say $N_p(t)$ ) of solutions over a finite field with $p\geq 3$ elements, then we have
$N_p(t)=N_p(16/t),$
provided $t$ is not in the set $\{0, 4, -4\}$ . This was a fact that Fouvry, Michel and myself found out when working on our paper on algebraic twists of modular forms.

At the time, I knew one proof, based on computations with Magma: the curves above “are” elliptic curves, and Magma found an isogeny between the curves with parameters $t$ and $16/t$ , which implies that they have the same number of points by elementary properties of elliptic curves over finite fields.

By now, however, I am aware of three other proofs:

The most elementary, which motivates this post, was just recently put on arXiv by T. Budzinski and G. Lucchini Arteche; it is based on the methods of Chevalley’s proof of Warning’s theorem: it computes $N_p(t)$ modulo $p$ , proving the desired identity modulo $p$ , and then concludes using upper bounds for the number of solutions, and for its parity, to show that this is sufficient to have the equality as integers. Interestingly, this proof was found with the help of high-school students participating in the Tournoi Français des Jeunes Mathématiciennes et Mathématiciens. This is a French mathematical contest for high-school students, created by D. Zmiaikou in 2009, which is devised to look much more like a “real” research experience than the Olympiads: groups of students work together with mentors on quite “open-ended” questions, where sometimes the answer is not clear (see for instance the 2016 problems here).
A proof using modular functions was found by D. Zywina, who sent it to me shortly after the first post (I have to look for it in my archives…)
Maybe the most elegant argument comes by applying a more general result of Deligne and Flicker on local systems of rank $2$ on the projective line minus four points with unipotent tame ramification at the four missing points (the first cohomology of the curves provide such a local system, the missing points being $0, 4, -4, \infty$ ). Deligne and Flicker prove (Section 7 of their paper, esp. Prop. 7.6), using a very cute game with products of matrices and invariant theory, that if $\mathcal{V}$ is such a local system and $\sigma$ is any automorphism of the projective line that permutes the four points, then $\sigma^*\mathcal{V}$ is isomorphic to $\mathcal{V}$ .

Not too bad a track record for such a simple-looking question… Whether there is a bijective proof remains open, however!

Horizon

The Swiss Science Foundation (SNF in German, FNS in French) publishes a regular magazine that surveys current topics concerning science and research in Switzerland. It often highlights subjects that are unexpected and interesting in all areas of science, from the humanities to forestry, the deterioration of prussian blue in paintings and so on, and does so in its three parallel editions, in English, French and German.

The last issue has a special focus on Open Science in its various forms. For some reason, although there is no discussion of Polymath per se, the editors decided to have a picture of a mathematician working on Polymath as an illustration, and they asked me if they could make such a picture with me, and in fact two of them (the photographer is Valérie Chételat) appear in the magazine. Readers may find it amusing to identify which particular comment of the Polymath 8 blog I am feigning to be studying in those pictures…

Besides (and of greater import than) this, I recommend looking at the illustration pages 6 and 7,

which is a remarkably precise computer representation of the 44000 trees in a forest near Baden, each identified and color-coded according to its species… (This is done by the team of M. Schaepman at the University of Zürich).