It was almost twenty years ago that I started drawing and looking at the graphs of Kloosterman sums (at least, that’s a likely date; I don’t remember when it began, but I put some drawings as whimsical illustrations — see, e.g., page 26 — in my PhD thesis to enliven it, and that was around 1997–1998).
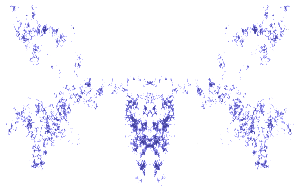
This year, by a lucky coincidence, I can finally say something definite about these graphs, and indeed something quite interesting. What happened is that Will Sawin was visiting Switzerland in June to talk to Philippe Michel and me about some very nice results about trace functions, and he noticed a picture like the one above that I have on the whiteboard next to my office. He asked me what it was, and more or less immediately it became clear that we could try to determine, for instance, the distribution of the “middle point” (corresponding to the partial sum up to ). Then, in a few more hours, we realized that we should be able to go much further. After a few more months, I’ve just uploaded to arXiv the paper we wrote about this subject, which is also available on my web page. It is a combination of probability, algebraic geometry and analytic number theory which I personally find extremely appealing and pleasant.
In rough terms, what we show is that, if one normalizes the Kloosterman paths properly, as continuous maps , and consider those paths associated to all Kloosterman sums modulo a large prime
, then these will, statistically, “look like” the graphs
of a certain very specific random Fourier series.
Precisely, for a prime , an invertible residue class
modulo
and a real number
, let’s denote by
the point at “time” on the graph joining the partial sums of
each segment being parameterized linearly by an interval of length . This corresponds to normalizing the pictures I used to draw by saying that they correspond to a parameterization by
, and also by scaling by the factor
, which has the effect that the endpoint is always a real number in
, by the Weil bound for Kloosterman sums.
We interpret the data as a stochastic process; the “randomness” is in the residue class
, which is supposed to be chosen uniformly at random among invertible classes modulo
.
Our first result is convergence in the sense of finite distributions: we consider any fixed finite number of “times” , and prove that the random vectors
have a limit, in the sense of convergence in distribution, as tends to infinity.
This limit is explicit: it is
where is a very nice (a.s.) continuous stochastic process, defined in the following manner: take any sequence
of independent random variables which are all Sato-Tate distributed, and put
a random Fourier series (here the term has to be interpreted as
).
Remark. It would be interesting to know if this series has already appeared in other contexts; our searches of the literature on random Fourier series have not yet found any previous occurence.
The proof is actually relatively short, but nevertheless this is a very deep property. Indeed, it depends in an essential way on Deligne's Riemann Hypothesis over finite fields, and on the computation of the monodromy groups of Kloosterman sheaves by Katz.
In fact, it is a very interesting instance where the application of the Riemann Hypothesis requires not only to understand when it gives cancellation in exponential sums, but also requires in an essential way the knowledge of the main term when there is no cancellation. This seems (to me at least) to require in an essential way the group-theoretic interpretation of the equidistribution of Kloosterman sums with respect to the Sato-Tate measure, because it is this interpretation that allows us to control arbitrary moments of partial sums of Kloosterman sums using the Goursat-Kolchin-Ribet criterion from group theory (roughly speaking, an algebraic subgroup of that surjects to each factor is the whole product group).
Remark. As a simple consequence, note that is Sato-Tate distributed, so this result contains the “vertical” Sato-Tate law of Katz.
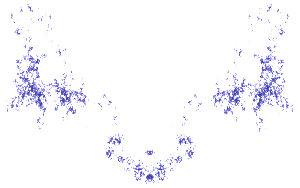
Plotting some samples of this random Fourier series, by interpolating linearly between the values of samples of partial sums, we get pictures with a definite air de famille:
There is an obvious question that arises from this result, which probabilists will already have raised: since both our stochastic processes and the limit
are continuous processes, we should really be trying to prove convergence in the space of continuous processes, i.e, when viewing the Kloosterman paths as random variables with values in the Banach space of continuous functions on
.
We tried to do this. Using Kolmogorov’s criterion for tightness (and the previous result), one can see that the question is intimately related to non-trivial (average) estimates for short partial sums of Kloosterman sums just around the Pólya-Vinogradov range. We have not (yet) established the required estimate, which seem quite delicate, but it is very interesting to see a unification of two aspects of exponential sums which are extremely important in applications, namely equidistribution of Katz-Sato-Tate type, and estimate for short sums (of length about square root of the number of terms). Moreover, using this approach, we show easily that further average over the additive character defining Kloosterman sums does lead to convergence to as continuous processes. As a first application, we get a rather elementary proof of tail bounds for the supremum of the partial sums (with this extra average), using some nice facts about probability in Banach spaces (I may write a bit about this in a later post).
I talked about this result in Oxford a few weeks ago, on the occasion of a workshop on analytic number theory there (this might have been one of the first mathematical talks coming with its own app!).
We have dedicated this paper to the memory of Marc Yor.
You know, this “Goursat-Kolchin-Ribet criterion” comes up again and again (if I understand what you mean by it) and is very handy. Dunfield and Thurston call it (in its finite group manifestation) Hall’s lemma and trace it back to a 1937 paper of (Philip) Hall.
Yes, it’s the same, and I remember also being amused to see it mentioned by this name in the paper of Dunfield and Thurston!