I am currently the head of the Mathematics Library at ETH (which is separate from the main library). A few days ago, I surveyed some of the (relatively) old books in our collection with one of the librarians, just to see if some of these should be handled in a special way. We didn’t find anything really out of the ordinary (no copy of Poincaré’s works heavily annotated by H. Weyl, I’m afraid), but one book has some historical interest: it is (or seems to be) Stickelberger’s copy of Jacobi’s “Canon arithmeticus”
a table of primitive roots and discrete logarithms for primes up to 1000.
Stickelberger’s signature is found on one of the first pages
The table itself, as it took me a few minutes to understand (my Latin being non-existent), lists for each prime the “Numeri”
and the “Index”
, which are defined by the relation
for some primitive root modulo
, which can be identified easily by looking for the number for which
is equal to
:
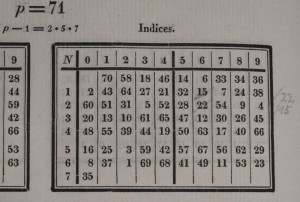
So above we see that Jacobi selected as primitive root modulo
and
, and
as primitive root modulo
, or
as primitive root modulo
. Obligingly, he also indicates the factorization of
(so that all primitive roots can be easily found by checking whether the corresponding index is coprime with
).
Like the copy which was digitized by Google, Stickelberger’s has a list of corrections at the end, and most (if not all: I didn’t check…) of these are incorporated in pencil in the main text, as here with :
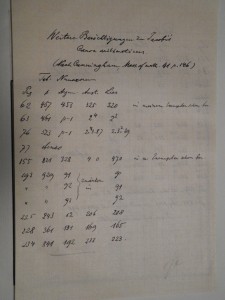
However, Stickelberger (if it was him) also had another list of corrections, written down on a separate loose sheet of paper inserted at the end of the book.
These corrections are reproduced from the paper On quasi-mersennian numbers by Lieutenant Colonel Allan Cunningham in Vol. 41 of the Messenger of Mathematics (a volume which seems famous in statistical circles because it contains, ten pages later, an important paper of R.A. Fisher on maximum likelihood…) But even Cunningham’s corrections contain a few mistakes, which Stickelberger reports (though with question marks):
Indeed, for , the primitive root chosen by Jacobi is
and we have
instead of reported by Cunningham (and
in the Canon).
As far as I could see during my quick inspection, there are no further annotations or comments by Stickelberger, nor any date indicating when he acquired this book. The publication date is 1839, and the only other indication is that the volume of the Messenger of Mathematics with Cunningham’s paper appeared in 1912. I also do not known when and how the book entered the collection of ETH.

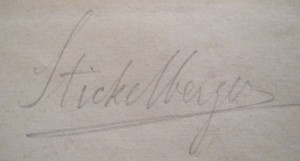
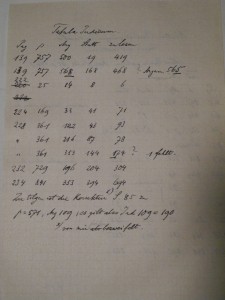
Interesting. You should change “Stickelberg” to “Stickelberger” in the main body of the text.
Thanks for the correction!