France – Page 2 – E. Kowalski's blog

Update on a bijective challenge

A long time ago, I presented in a post a fun property of the family of curves given by the equations
$x+1/x+y+1/y=t,$
where $t$ is the parameter: if we consider the number (say $N_p(t)$ ) of solutions over a finite field with $p\geq 3$ elements, then we have
$N_p(t)=N_p(16/t),$
provided $t$ is not in the set $\{0, 4, -4\}$ . This was a fact that Fouvry, Michel and myself found out when working on our paper on algebraic twists of modular forms.

At the time, I knew one proof, based on computations with Magma: the curves above “are” elliptic curves, and Magma found an isogeny between the curves with parameters $t$ and $16/t$ , which implies that they have the same number of points by elementary properties of elliptic curves over finite fields.

By now, however, I am aware of three other proofs:

The most elementary, which motivates this post, was just recently put on arXiv by T. Budzinski and G. Lucchini Arteche; it is based on the methods of Chevalley’s proof of Warning’s theorem: it computes $N_p(t)$ modulo $p$ , proving the desired identity modulo $p$ , and then concludes using upper bounds for the number of solutions, and for its parity, to show that this is sufficient to have the equality as integers. Interestingly, this proof was found with the help of high-school students participating in the Tournoi Français des Jeunes Mathématiciennes et Mathématiciens. This is a French mathematical contest for high-school students, created by D. Zmiaikou in 2009, which is devised to look much more like a “real” research experience than the Olympiads: groups of students work together with mentors on quite “open-ended” questions, where sometimes the answer is not clear (see for instance the 2016 problems here).
A proof using modular functions was found by D. Zywina, who sent it to me shortly after the first post (I have to look for it in my archives…)
Maybe the most elegant argument comes by applying a more general result of Deligne and Flicker on local systems of rank $2$ on the projective line minus four points with unipotent tame ramification at the four missing points (the first cohomology of the curves provide such a local system, the missing points being $0, 4, -4, \infty$ ). Deligne and Flicker prove (Section 7 of their paper, esp. Prop. 7.6), using a very cute game with products of matrices and invariant theory, that if $\mathcal{V}$ is such a local system and $\sigma$ is any automorphism of the projective line that permutes the four points, then $\sigma^*\mathcal{V}$ is isomorphic to $\mathcal{V}$ .

Not too bad a track record for such a simple-looking question… Whether there is a bijective proof remains open, however!

Reading spines up or down?

Among the minor cultural differences that separate countries is the question of the orientation of the text on the spine of books that identify their title and author when conveniently packed on book shelves: going up

proudly as French and German books, or going down

as English or American or Italian books? When books are ordered by topic or author, this leads to rather uncomfortable switches of orientation of the head as one scans bookshelves for the right oeuvre to read during a lazy afternoon.

Actually, these are more or less contemporary examples, and it seems that these conventions change with time. For instance, I have an old English paperback from 1951 where the title goes up instead of down:

Another from 1962 goes down. When did the change happen? And why? And how do other languages stack up? Is it rather a country-based preference? Are the titles of Italian-language books printed in Switzerland going up (like the French and German ones do), or down? And does this affect the direction in which shivers run along your spine when reading a scary story of murdered baronets in abandoned ruins?

(There’s of course the solution, admittedly snobbish, of writing the title and author’s name horizontally

as the Pléiade does, for instance).

Worms, brains and “graphes expanseurs”

Two days ago, I was in Paris and visited the École Polytechnique there (for the first time, as it turns out), in order to give the traditional introductory lecture presenting various aspects of mathematics that is given each year to the incoming class of students. (Many people would wonder how a new class can be incoming in April; this is because the students of École Polytechnique, which is a military school, begin their studies with a military/civil service from September to April).

I had selected expander graphs as the topic of my talk, as being simultaneously a very modern subject, that can be presented with very basic definitions (most students, coming from the French “Classes préparatoires” have had two very solid years of mathematical studies, but of a rather traditional kind), and that has links to many different areas, including more applied ones.

The slides of my presentation can be found here, in French, and I prepared an English translation there (up to the handwritten bits of information on certain pictures, which will remain in French…) Actually, the slides by themselves are not particularly interesting, since there are many remarks and details that I only described during the talk.

The talk begins with a discussion of graphs, continues with basic notions of expansion, and then defines expander graphs, with a bit of the history (especially the paper of Barzdin and Kolmogorov that I like very much), and concludes with two applications (among many…): Apollonian circle packings, and the Gromov-Guth knot-distorsion theorem. If this looks like a lot of ground to cover, this is because the talk was 1h30 long…

While preparing the first part of the talk, I researched some examples of graphs with more care than I had done before. Some of the things I found are extremely interesting, and since they might not be so well-known among my readers, I will present them quickly.

(1) The worm is Caenorhabditis elegans, more chummily known as C. elegans, one of the stars of neurosciences, as being the only animal whose entire nervous system has been mapped at the level of all individual neurons and connections between them. This was done in 1986 by White, Southgate, Thomson and Brenner, with minor corrections since then, and an important update and representation in 2011 by Varshney, Chen, Paniagua and Chklovskii. The resulting graph has 302 neurons (this number is apparently constant over all individuals), and about 8000 edges. (Interestingly, it is naturally a mix of directed and un-directed edges, depending on the type of biological connection). The paper of Varshney, Chen, Paniagua and Chklovskii is quite fascinating, as it investigates many mathematical invariants of the graph, and for instance compares the number of small subgraphs of various types with the expected number for random graphs with comparable parameters (certain subgraphs arise with higher frequency…)

Much more about C. elegans is found on dedicated websites, including Openworm, which seems to have as a goal to recreate the animal virtually…

(2) The brain is the humain brain; it can’t be mapped at the level of C. elegans (there are about 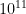$10^{11}$ neurons and 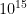$10^{15}$ synapses, from what I’ve seen), but I read a very interesting survey by Valiant about attempts to understand the computational model underpinning the reasoning capacities of the brain. He presents four basic tasks that must be among those that the brain performs, and explains how he succeeded in earlier work (from 1994 to 2005) in finding realistic algorithms and models for these problems. He comments:

In [5,14] it is shown that algorithms for the four random
access tasks described above can be performed on the
neuroidal model with realistic values of the numerical
parameters. The algorithms used are all of the vicinal
style. Their basic steps are all local in that they only
change synaptic strengths between pairs of neurons that
are directly connected. Yet they need to achieve the more
global objectives of random access. In order that they be
able to do this certain graph theoretic connectivity prop-
erties are required of the network. The property of
expansion [15], that any set of a certain number of
neurons have between them substantially more neighbors
than their own number, is an archetypal such property.
(This property, widely studied in computer science, was
apparently first discussed in a neuroscience setting [16].)
The vicinal algorithms for the four tasks considered here
need some such connectivity properties. In each case
random graphs with appropriate realistic parameters have
it, but pure randomness is not necessarily essential.

Here reference [16] is to the Barzdin-Kolmogorov paper.

(3) Lastly, I was wondering what is the size (number of vertices) of the largest graphs for which the first non-zero Laplace eigenvalue has been computed, approximately. The best I found is a paper of Kang, Breed, Papalexakis, and Faloutsos, which describes an algorithm that they have applied successfully to real-world graphs with about a billion vertices. This is rather impressive…

Radio

For those readers who understand spoken French (or simply appreciate the musicality of the language) and are interested in the history of mathematics, I warmly recommend listening to the recording of a recent programme of Radio France Internationale entitled “Pourquoi Bourbaki ?” In addition to the dialogue of Sophie Joubert with Michèle Audin and Antoine Chambert-Loir, one can hear some extracts of older émissions with L. Schwartz, A. Weil, H. Cartan, J. Dieudonné, for instance.

Proust, my family and Australia

When I was reading Proust, I noted with some amusement the character named simply “Ski” in the first volume of his appearance, a sculptor and amateur musician who is later revealed to be properly called “Viradobetski”, an actual name which was too complicated for the dear Madame Verdurin to try to remember. I just learnt from my better educated brother that

Proust s’est inspiré d’Henri Kowalski né en 1841, fils d’un officier polonais émigré en Bretagne. Il était à la fois compositeur de musique et concertiste.

Proust used as model Henri Kowalski, born in 1841, son of a Polish officer who emigrated to Brittany. He was a composer as well as a concert player.

to quote an authoritative list of Proust characters.

That Henri Kowalski is, it turns out, the son of Nepomus Adam Louis Kowalski, whose brother was Joachim Gabriel Kowalski, one of whose sons was Eugène Joseph Ange Kowalski, one of whose sons was Louis André Marie Joseph Kowalski, the fourth son of whom was my father. This puts me at genealogical distance (at most) six to a character from Proust. (Of course, rumors that Viradobetski was inspired by someone else can be safely discarded).

Wikipedia has a small page on Henri Kowalski, who was quite active and successful as a musician, and a rather impressive traveller. He spent thirteen years in Australia, leaving enough traces to be the subject of public lectures at the university of Melbourne. There are a few of his pieces on Youtube, for instance here. He also wrote a travel book which I now intend to read…